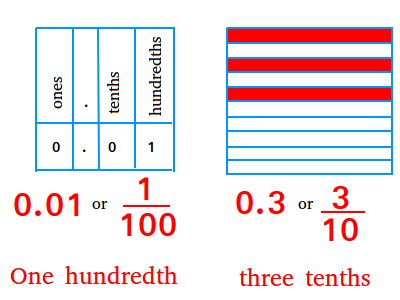
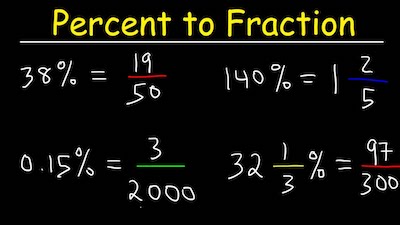Many math students struggle with conversions between percents, fractions, and decimals. However, these conversions don’t have to be difficult. In fact, they are quite easy. All it requires is for you to understand the concepts and the methods used. Then, it will be a matter of practicing just like everything else in math.
Learn everything you need to know about calculating fractions.
Converting Between Percents, Fractions, And Decimals
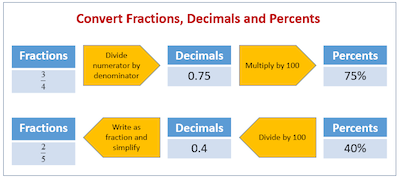
Simply put, percents, fractions, and decimals are just different ways of writing the same number. Here is a simple example:
Percent Fraction Decimal
50% 1/2 0.5
These are all equivalent. This means that in conversation, you may say that Anne ate 50% of the pizza, or 1/2 of the pizza, or 0.5 of the pizza. All three of these phrases mean the exact same thing.
#1: Converting Between Percents And Fractions:
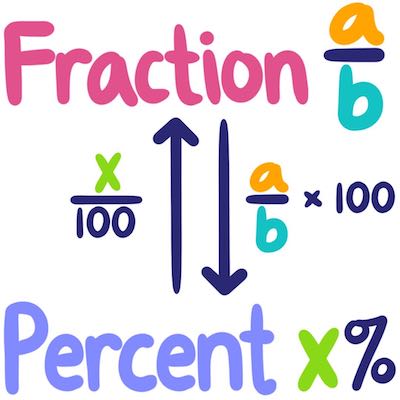
Percents To Fractions:
Percent-to-fraction conversions aren’t hard. This is where you use the fact that “percent” means “out of a hundred”. so, first, you convert the percent to a decimal, and then you convert the decimal to an out-of-a-hundred fraction. Then you simplify, if possible. For instance:
40% = 0.40 = 40/100
First, w dropped the “%” character and moved the decimal point two places to the left. Then you converted the decimal to an out-of-a-hundred fraction. Now you can reduce the fraction:
40/100 = 4/10 = 2/5
Most of these conversions are simple like the one above, but some require a little extra care. The reason we converted to a decimal first is that the number of decimal places tells me how many zeroes to have underneath. Notice that “0.40” can also be written as “0.4”. Then 0.4 = 4/10 = 2/5 , which is the same answer as before. It works out because “0.4” has one decimal place and “10” has one zero. This concept (matching the number of decimal places with the number of zeroes) helps in more complicated problems such as the following one:
104% = 1.04 = 104/100 = 26/25
Here are some more examples:
0.5% = 0.005 =5/1000 = 1/200
Confirm your results with our percent to fraction calculator.
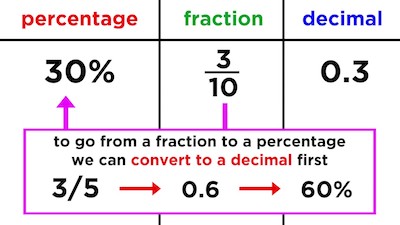
Fractions To Percents
Let’s convert 3/5 to a percent. The key here is to turn 3/5 into a fraction with a denominator of 100. To do this, we need to know what times 5 gives us 100:
5 X ? = 100
The number is 100 ÷ 5= 20:
5 X 20 = 100
Now we’re ready to convert 3/5 to a percent:
Step #1: Multiply to get a denominator or 100:
3/5 = (3 X 20) / (5 X 20)
Step #2: Simplify:
60/100
Step #3: Write as a percent:
60%
We figured out that 3/5 is equivalent to 60%.
Check out our online fraction to percentage calculator.
#2: Converting Between Percents And Decimals:
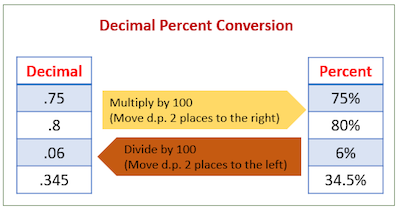
Percents To Decimals:
Let’s convert 8% to a decimal:
Step #1: Write the percent as a fraction:
8% = 8/100 = 0.08
We figured out that 8% is equivalent to 0.08.
Decimals To Percents:
Let’s convert 0.05 to a percent:
0.05 = 5/100
Write as a percent:
5%
We figured out that 0.05 is equivalent to 5%.
Discover how to calculate decimal to fraction using our free calculator.
Common Fractions With Decimal And Percent Equivalents
| Fraction | Decimal | Percent |
| 1/2 | 0.5 | 50% |
| 1/3 | 0.33333 | 33.333% |
| 2/3 | 0.66666 | 66.6666% |
| 1/4 | 0.25 | 25% |
| 3/4 | 0.75 | 75% |
| 1/5 | 0.2 | 20% |
| 2/5 | 0.4 | 40% |
| 3/5 | 0.6 | 60% |
| 4/5 | 0.8 | 80% |
| 1/6 | 0.16666 | 16.666% |
| 5/6 | 0.83333 | 83.333% |
| 1/8 | 0.125 | 12.5% |
| 3/8 | 0.375 | 37.5% |
| 5/8 | 0.625 | 62.5% |
| 7/8 | 0.875 | 87.5% |
| 1/9 | 0.111 | 11.111% |
| 2/9 | 0.222 | 22.222% |
| 4/9 | 0.444 | 44.444% |
| 5/9 | 0.555 | 55.555% |
| 7/9 | 0.777 | 77.777% |
| 8/9 | 0.888 | 88.888% |
| 1/10 | 0.1 | 10% |
| 1/12 | 0.08333 | 8.333% |
| 1/16 | 0.0625 | 6.25% |
| 1/32 | 0.03125 | 3.125% |