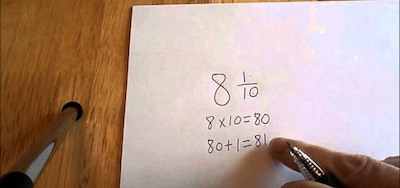a / b + c / d | a b / c + d e / f | |||
| Answer: | ||
| Result= | ||
From our experience, a lot of students have a lot of problems when then need to solve problems that include adding fractions.
The truth is that adding fractions isn’t difficult but you need to understand the process.
However, when you are simply looking to confirm your results or you need a quick fraction addition, then the best thing you can do is to use our fraction calculator.
Using Our Fraction Calculator

If you take a look at our fraction calculator, you can easily see that it is very versatile. After all, you will be able to choose the type of fractions that you are looking to add. As soon as you do this, you will just need to add the numerators and denominators to the specific fields, and click on the calculate button.
But to ensure that you know how to use it without any problems, let’s check a couple of examples using both types of fractions additions.
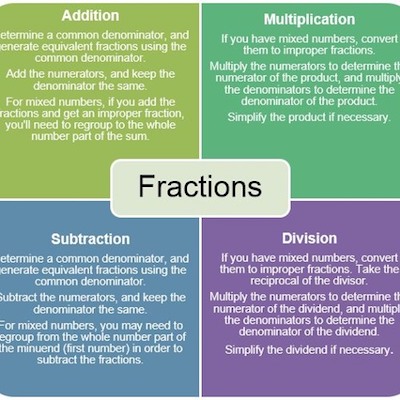
Looking to subtract fractions?
#1: Adding Fractions Such As (a/b) + (c/d)
Example #1: Let’s imagine that you are trying to add the fractions 2/3 and 1/8.
As we have already mentioned above, in order to use our calculator with fraction, you will need to add the respective numerators and denominators of the fractions to their respective fields. So, as soon as you add the numbers and click on the Calculate button, you will get the result. In this case, the result is: 19/24. It’s important to notice that right below the fraction result, you will also be able to see the result in decimals. So, in this case:
(2/3) + (1/8) = (19/34) = 0.79
Discover how to divide fractions.
Example #2: Let’s now say that you are trying to add the fractions 3/4 and 3/8.

As we have already mentioned above, in order to use our calculator with fraction, you will need to add the respective numerators and denominators of the fractions to their respective fields. So, as soon as you add the numbers and click on the Calculate button, you will get the result. In this case, the result is: 1 (1/8). It’s important to notice that right below the fraction result, you will also be able to see the result in decimals. So, in this case:
(3/4) + (3/8) = 1 (1/8) = 1.13
One of the things that you may not have noticed in our calculator with fraction is that you have an arrow in the middle of the fractions. Well, if you click on this arrow, you will be able to choose the math operation that you want to use with these fractions. So, as you can see, our fraction addition calculator is a lot more versatile than you could think at first glance. After all, you can not only add fractions but you can also subtract fractions, multiply fractions, and even divide fractions.
Learn a bit more about fractions here.
#2: Adding Fractions Such As a (b/c) + d (e/f)
Example #1: Let’s imagine that you are trying to add the fractions 4 (1/3) and 5 (6/7).
As we have already mentioned above, in order to use our calculator with fraction, you will need to add the respective numerators and denominators of the fractions to their respective fields. So, as soon as you add the numbers and click on the Calculate button, you will get the result. In this case, the result is: 10 (4/21). It’s important to notice that right below the fraction result, you will also be able to see the result in decimals. So, in this case:
4 (1/3) + 5 (6/7) = 10 (4/21) = 10.19
Having problems multiplying fractions?
Example #2: Let’s now say that you are trying to add the fractions 2 (3/4) and 3 (3/8).

As we have already mentioned above, in order to use our calculator with fraction, you will need to add the respective numerators and denominators of the fractions to their respective fields. So, as soon as you add the numbers and click on the Calculate button, you will get the result. In this case, the result is: 6 (1/8). It’s important to notice that right below the fraction result, you will also be able to see the result in decimals. So, in this case:
2 (3/4) + 3 (3/8) = 6 (1/8) = 6.13
Again, and also in this type of fractions, you can see that you also have the option not only to add fractions but also to subtract, multiply, and divide them.
While using our fraction addition calculator makes all the calculations extremely simply, it is important that you know how to add fractions by hand. So, this is what we are going to show you.
Discover how to use our mixed fraction calculator.
Adding Fractions By Hand
When you are trying to figure out how you can learn how to add fractions by hand, the first thing that you need to do is to look at the fractions that you want to add. Here, you will have two different cases or situations:
#1: You Want To Add Fractions With The Same Denominator:
When you want to add two fractions that have the same denominator, all you need to do is to add the numerators and maintain the common denominator. As soon as you do this, you should try to simplify the fraction to the lowest terms.
Let’s check a couple of examples to make sure that you understand.
Example #1: Imagine that you want to add 5/7 and 6/7.
As you can see, these fractions have a different numerator (5 and 6) but the denominator is the same – 7. As we already mentioned, in this case, you will need to add the numerators and simply leave the denominator unchanged. This means that:
5/7 + 6/7 = (5 + 6)/7 = 11/7
Example #2: Imagine that you want to add 3/8 and 1/8.
As you can see, these fractions have a different numerator (3 and 1) but the denominator is the same – 8. As we already mentioned, in this case, you will need to add the numerators and simply leave the denominator unchanged. This means that:
3/8 + 1/8 = (3 + 1)/8 = 4/8
As you can easily see, it is possible to simplify this fraction. After all, f you divide the fraction by 4 (both the numerator and denominator), you will get:
4/8 = 1/4
#2: You Want To Add Fractions With A Different Denominator:

Up until now, we showed you how to add the simplest fractions – the ones that have the same denominator. However, it is time to turn things a bit more interesting. So, you are about to learn how you can add fractions with different denominators.
When you are looking to add fractions that have a different denominator, before adding fractions together, you need to have like denominators. One of the easiest ways to do this is o simply multiply the first denominator by the second one and vice-versa. Notice that when you are doing this, you will also need to multiply the numerators as well. In case you don’t, you will end up with a different value.
While this may seem a bit confusing, it really isn’t. Let’s check some examples so that you can understand the entire process.
Example #1: Let’s say that you want to add 2/3 and 2/4.
As you can see, in this case, the denominators are different – 3 and 4. So, the first thing that you will need to do is to convert the fractions to get common denominators.
Just take a look at the denominators and multiply each by the other denominator. Besides, don’t forget that you also need to multiply the numerator by the same number as its denominator to keep the value the same.
In this particular case, you will need to multiply the first fraction (2/3) by 4 and the second fraction (2/4) by 3:
[ (2 x 4) / (3 x 4) ] + [ (2 x 3) / (4 x 3) ] = (8/12) + (6/12)
Now, you already have a common denominator. So, you just need to follow the rule that we already explained to you that says that you just need to add the numerators while maintaining the denominator. So:
(8/12) + (6/12) = (8 + 6) /12 = 14/12
The final solution is an improper fraction, so you need to convert it to a mixed number and simplify the solution:
1 (2/12)
By dividing the numerator and denominator by 2, you can simplify the solution to:
1 (1/6)
Example #2: Let’s say that you want to add 5/6 and 7/10.
As you can see, in this case, the denominators are different – 6 and 10. So, the first thing that you will need to do is to convert the fractions to get common denominators.
Just take a look at the denominators and multiply each by the other denominator. Besides, don’t forget that you also need to multiply the numerator by the same number as its denominator to keep the value the same.
In this particular case, you will need to multiply the first fraction (5/6) by 10 and the second fraction (7/10) by 6:
[ (5 x 10) / (6 x 10) ] + [ (7 x 6) / (10 x 6) ] = (50/60) + (42/60)
Now, you already have a common denominator. So, you just need to follow the rule that we already explained to you that says that you just need to add the numerators while maintaining the denominator. So:
(50/60) + (42/60) = (50+ 42) / 60 = 92/60
The final solution is an improper fraction, so you need to convert it to a mixed number and simplify the solution:
1 (32/60)
By dividing the numerator and denominator by 2, you can simplify the solution to:
1 (8/15)
How to convert a fraction into a decimal?
#3: You Want To Add Fractions With Mixed Numbers and Improper Fractions:
In case you don’t know, a mixed number is one that contains a fraction as well as a whole number, such as:
5 (3/4), 3 (7/10), 6 (7/8)
Adding these numbers can be a bit more difficult.
So, to ensure that we keep the process as simple as we can, you should start by converting the number to an improper fraction.
In case you don’t know, an improper fraction has a numerator that is larger than the denominator. Because of this, it’s important to simplify the fraction after finding the solution since you’ll probably end up with another mixed number.
Let’s take a look at an example.
Discover how to convert a decimal into a fraction.
Example: Imagine that you want to add the fractions 2 (3/4) and 1/4.
In this equation, you know that:
1 = 4/4
Therefore:
2 = 8/4.
So, add the fraction to the converted whole number:
[(8 + 3) / 4] + (1/4) = 11/4 + 1/4
Since we now already have the same denominator in both fractions, you just need to add the numerators and maintain the denominator:
11/4 + 1/4 = 12/4 = 3
Discover all the rules for fractions.
Conclusion

As you can see, adding fractions isn’t very hard, The secret is to understand the process that you need to follow for each one the situations. As soon as you get the fractions to have the same denominator, you will just need to add the numerators and maintain the denominator.
As soon as you complete the calculations, you want to make sure that you can’t simplify the fractions. After all, this is when your exercise will be marked as complete.