While many people don’t like math, it is impossible to deny that math is a part of our daily lives. No matter if you are talking about fractions, decimals, ratios, and percentages, they are all a part of your life. Just think of when you go shopping, when you are budgeting, interpreting odds and probabilities, diluting mixtures, converting among metric units, understanding scales on maps, among so many others.
Looking to know more about fractions?
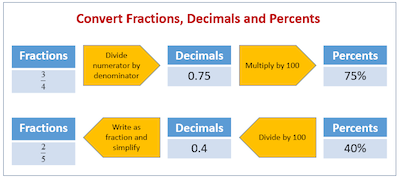
One of the main ideas that you should keep in mind is that when you have a good grasp of fractions, decimals, ratios, and percentages, then you are ready to deal with more complex math topics including fancy algebra, coordinate geometry, trigonometry, among others.
Understanding The Part/Whole Concept
While you may have not noticed, you keep using fractions on your day. Just think of the bath being two thirds full, the third quarter of the netball game, half an hour, or even one-quarter of a cup of sugar.
Tale a look at our convert percent to fraction calculator.
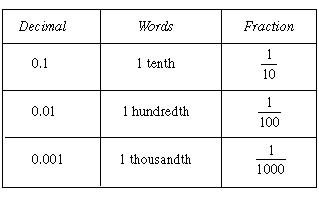
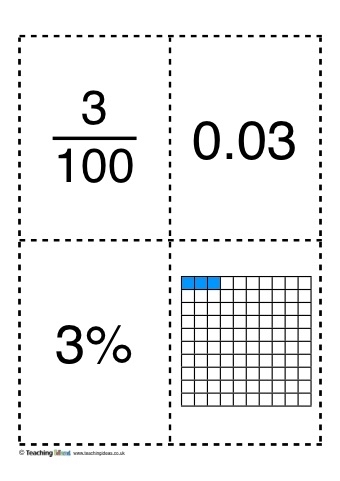
As you can now understand, the part/whole concept is present in fractions that can be expressed as a/b and that are used when a part is being compared to the whole.
As you probably already know, the a in the fraction is called the numerator and the b is called the denominator. We can then state that the numerator means to the number of pieces of the whole lot, and the denominator signifies the number of pieces of equal size into which the whole is divided.
Let’s take a look at some examples so that you can fully understand this part/whole concept.
Example #1:
Let’s say that you have $5 and that you want to share it between person A and person B. Person A gets $3 and person B gets $2.
Based on this division, you can state that person A’s share is three fifths (3/5) of the whole ($5) and person B’s share is two fifths (2/5) of the whole ($5).
Make sure to use our free percentage to fraction calculator.
Example #2:
Imagine that you have a class of 60 students where 36 are females and 24 are males.
Based on this knowledge, you can then state that thirty-six out of sixty (36/60) students are females, and twenty-four out of sixty (24/60) are males.
The two fractions can be read as thirty-six sixtieths and twenty~four sixtieths.
This part/whole concept can be represented in a diagram:
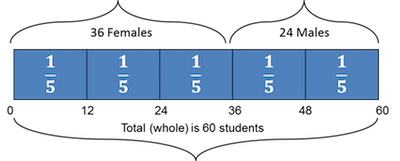
If you look at both fractions of the example, a common factor of both the numbers, thirty-six (36) and twenty-four (24) is twelve (12). Twelve is one fifth (1/5) of the total 60, as five times twelve is sixty (5 x 12 = 60). 5 is the lowest common denominator for these given fractions.
Having doubts? Confirm your results with our percent to fraction calculator.
But you can also say that thirty-six out of sixty (36/60) students are females and twenty-four out of sixty (24/60) are males. This means that three out of five (3/5) students are females and two out of five (2/5) students are males. The fractions 3/5 and 2/5 are read as three fifths and two fifths, respectively.